


Next: Estimation of the two-loop
Up: Statistical Description of Acoustic
Previous: Rules for writing and
Let us start from (4.18) and
introduce
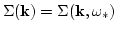 with
with
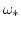 given by (4.14):
given by (4.14):
where
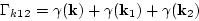 |
(113) |
is ``triad-interaction'' frequency and
 is triad
interaction time. One can consider (B1 - B2) as an
integral equation for the damping of wave
is triad
interaction time. One can consider (B1 - B2) as an
integral equation for the damping of wave
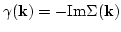 and for the frequency
and for the frequency
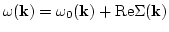 .
.
First we consider these equations in the limit of weak interaction
where, 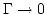 , and the main contribution to the first term in
(B1) comes from the region where
, and the main contribution to the first term in
(B1) comes from the region where
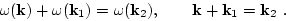 |
(114) |
These are conservation laws for 3-wave confluence processes 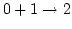 . The main contribution for the second term in (B1) comes
from the region
. The main contribution for the second term in (B1) comes
from the region
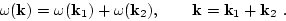 |
(115) |
These are conservation laws for decays processes 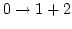 . For weak
interaction one may replace in (4.2) and (4.3)
. For weak
interaction one may replace in (4.2) and (4.3)
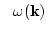 on
on
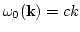 . Than it follows from
(4.2) and (4.3) that
. Than it follows from
(4.2) and (4.3) that
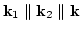 with
with
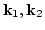 directed along
directed along 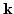 . This fact makes it natural to introduce in integrals (B1)
new variables: scale positive variable
. This fact makes it natural to introduce in integrals (B1)
new variables: scale positive variable  and two-dimensional
vector
and two-dimensional
vector 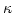 such that
such that
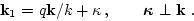 |
(116) |
In the first term of (B1)
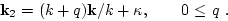 |
(117) |
In the second term
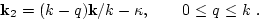 |
(118) |
For 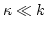 the denominators in integrals (B1) strongly
depend on
the denominators in integrals (B1) strongly
depend on 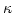 . Indeed:
. Indeed:
This allows to neglect 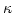 dependence of interaction
dependence of interaction
 and correlation
and correlation 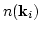 in numerator of
(B1) for estimation. The result is
in numerator of
(B1) for estimation. The result is
where
 |
(122) |
is a factor in (2.20) so that for parallel or almost parallel
wavevectors
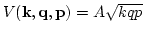 . After
changing of variables this integral becomes to be more transparent:
. After
changing of variables this integral becomes to be more transparent:
One may estimate
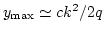 from the fact that
our expressions were obtained by expanding in
from the fact that
our expressions were obtained by expanding in 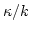 , therefore
should be at least
, therefore
should be at least 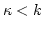 .
.
Now let us consider the imaginary and real part of  separately. It is convenient to begin with
separately. It is convenient to begin with
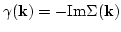 :
:
Here we changed the upper limit of integration:
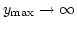 because the main contribution to the integral comes from the area
because the main contribution to the integral comes from the area
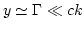 . After trivial integration with respect of
. After trivial integration with respect of 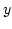 one has:
one has:
This expression for
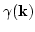 corresponds to that given by
the kinetic equation [1] for waves. For further progress it is
necessary to do some assumption about
corresponds to that given by
the kinetic equation [1] for waves. For further progress it is
necessary to do some assumption about 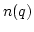 . Let us assume that
. Let us assume that
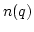 vanishes with growing of
vanishes with growing of 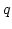 faster than
faster than
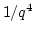 .
.![[*]](file:/usr/share/latex2html/icons/footnote.png) For such
spectra the main contribution to the integral comes from small
For such
spectra the main contribution to the integral comes from small 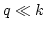 . In this case contributions from first and second integrals in
(B14) coincides and may be represented in the form:
. In this case contributions from first and second integrals in
(B14) coincides and may be represented in the form:
 |
|
|
(126) |
In this case contributions from first and second integrals in
(B14) coincides and may be represented in the form:
 |
|
|
(127) |



Next: Estimation of the two-loop
Up: Statistical Description of Acoustic
Previous: Rules for writing and
Dr Yuri V Lvov
2007-01-17
![]() , and the main contribution to the first term in
(B1) comes from the region where
, and the main contribution to the first term in
(B1) comes from the region where
![]() separately. It is convenient to begin with
separately. It is convenient to begin with
![]() :
: