Problems 1,2 and 3 are taken from the First Edition.
- For an infinitesimal volume, informally show that
where 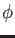 is some differentiable propery of fluid. Hence informally
deduce that
is some differentiable propery of fluid. Hence informally
deduce that
- Show that the derivative of an integral is given by
By generalizing to three dimensions show that the material derivative of an
integral of fluid property is given by
where the surface integral 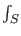 is over the surface bounding the volume
is over the surface bounding the volume
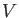 .
Hence show that
.
Hence show that
Hint You may find it useful to Google Leibnitz's rule and
Reynolds transport theorem.
- Why is there no diffusion term in the mass continuity equation?
Suppose that a fluid contains a binary mixture of dry air and water
vapour. Show that the change in mass of a parcel of air due to the
diffusion of water vapour is exactly balanced by the diffusion of
dry air in the opposite direction.
- Estimate the Reynolds number of flow generated by your car when you
go on the North way according to the speed limit. Assume that the
size of vortices generated by your car are of the order of the car
cross section, nad velocity of vortices are roughly equal to the
velocity of your car. The kinematic viscosity of air at 15
Centigrade is
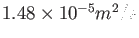 . Estimate the Reynolds numbers
in a water bottle that you rigorously shake, and on the commercial airplane
wing.
. Estimate the Reynolds numbers
in a water bottle that you rigorously shake, and on the commercial airplane
wing.
Dr Yuri V Lvov
2020-02-25
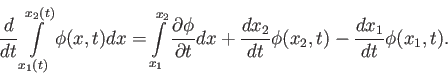
![\begin{displaymath}\frac{D}{D t} \int_V \phi(x,t) d V = \int \frac{\partial \phi...
...partial \phi}{\partial t} + \nabla\cdot({\bf v}\phi)\right]d V.\end{displaymath}](img9.png)