


Next: Hamiltonian of Acoustic Turbulence
Up: Hamiltonian Description of Acoustic
Previous: Hamiltonian Description of Acoustic
Consider again the Euler equations for a compressible fluid
(2.1). The enthalpy of a unit mass  is equal to the
derivative of internal energy of unit volume
is equal to the
derivative of internal energy of unit volume
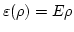 with respect to fluid density:
with respect to fluid density:
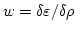 .
As a result of direct differentiation with respect to time, it is
readily evident that equations (2.1) conserve the energy of the
fluid
.
As a result of direct differentiation with respect to time, it is
readily evident that equations (2.1) conserve the energy of the
fluid
![\begin{displaymath}
{\cal H}=\int[\rho v^2/2+ \varepsilon(\rho)]\,d{\bf r}\ .
\end{displaymath}](img201.png) |
(33) |
One can show (and see for example[1]) that Eqs. (2.1) may
be written in the Hamiltonian form:
if the velocity
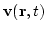 is presented in terms of two
pairs of Clebsch variables
is presented in terms of two
pairs of Clebsch variables 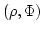 and
and
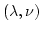 as follows,
as follows,
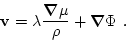 |
(36) |
Here the energy (2.11) is expressed in terms
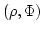 and
and
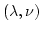 so that (2.14) becomes the Hamiltonian of the
system. As seen from (2.14), the case with
so that (2.14) becomes the Hamiltonian of the
system. As seen from (2.14), the case with 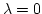 or
or
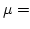 const corresponds to potential fluid motions which are defined
by a pair of variables (
const corresponds to potential fluid motions which are defined
by a pair of variables (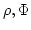 ) according to equations
(2.12). It is convenient to transform in the
) according to equations
(2.12). It is convenient to transform in the 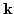 -representation from the real canonical variables,
-representation from the real canonical variables,
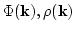 to the complex ones
to the complex ones 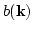 and
and
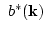 ,
,
Here
![$\delta\rho({\bf k})=[\rho({\bf k})-\rho_0({\bf k})]$](img220.png) is the
Fourier transform of density deviation from the steady state.
is the
Fourier transform of density deviation from the steady state.



Next: Hamiltonian of Acoustic Turbulence
Up: Hamiltonian Description of Acoustic
Previous: Hamiltonian Description of Acoustic
Dr Yuri V Lvov
2007-01-17